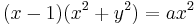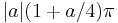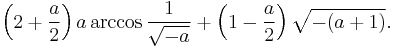Conchoid of de Sluze

The conchoid(s) of de Sluze is a family of plane curves studied in 1662 by René François Walter, baron de Sluze.

The curves are defined by the polar equation

$r=\sec\theta%2Ba\cos\theta \,$ .

In cartesian coordinates, the curves satisfy the implicit equation

$(x-1)(x^2%2By^2)=ax^2 \,$

except that for a=0 the implicit form has an acnode (0,0) not present in polar form.

These expressions have an asymptote x=1 (for a≠0). The point most distant from the asymptote is (1+a,0). (0,0) is a crunode for a<−1.

The area between the curve and the asymptote is, for 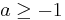$a \ge -1$ ,

$|a|(1%2Ba/4)\pi \,$

while for 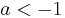$a < -1$ , the area is

$\left(1-\frac a2\right)\sqrt{-(a%2B1)}-a\left(2%2B\frac a2\right)\arcsin\frac1{\sqrt{-a}}.$

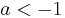If $a<-1$ , the curve will have a loop. The area of the loop is

$\left(2%2B\frac a2\right)a\arccos\frac1{\sqrt{-a}} %2B \left(1-\frac a2\right)\sqrt{-(a%2B1)}.$

Four of the family have names of their own:

a=0, line (asymptote to the rest of the family)